Electrophoresis

Introduction

Electrophoresis is a separations technique that is based on the the mobility of ions in an electric field. Positively charged ions migrate towards a negative electrode and negatively-charged ions migrate toward a positive electrode. For safety reasons one electrode is usually at ground and the other is biased positively or negatively. Ions have different migration rates depending on their total charge, size, and shape, and can therefore be separated.

General principle

An exact theory of electrophoresis seems almost impossible to develop. Serious difficulties arise because electrophoresis must almost always be carried out in aqueous solutions containing buffer ions and salts in addition to the macromolecule of interest; at the very least the counterions that have dissociated from the macromolecule to provide its charge must be present. To simplify the problem, we may write the basic equation for electrophoresis in exactly the same fashion as for sedimentation; the only difference is the source of force. The force experienced by a particle in an electrical field is given by Coulomb's law,

F = ZeE

where Z is the number of charge, e is the magnitude of the electronic charge, and E is the electrical field in units of potential per centimeter. Since the resistance to motion is given by -fv, where f is the frictional factor, and v the velocity, the condition that the net force on the particle be zero in steady in steady motion tells us:

fv = ZeE

The equation above can be rearranged to define an electrophoresis mobility U, a quantity very analogous to the sedimentation coefficient.

U = v/E = Ze/f

As with the sedimentation coefficient, U is the ratio of velocity to the strength of the driving field. If the particle happens to be spherical, Stroke's law applies and we can write

U = Ze/6phR

where R is the particle radius and h the solvent viscosity.

Some media for zonal electrophoresis
Medium	Conditions	Principle Uses
Paper	Filter paper moistened with buffer, placed between electrodes	Small molecules: amino acids or nucleotides
Polyacrylamide gel	Cast in tubes or slabs; cross-linked	Proteins and nucleics
Agarose gel	As polyacrylamide, but no cross-linking	Vary large proteins, nucleic acids, nucleoproteins. etc.

A macromolecule cannot move as easily through a network as ia can when free in solution. In fact, a very simple relationship has been discovered between relative mobility and gel concentration. Such graphs are called Ferguson plots:

log U_ri = log U⁰_ri - k_iC

where C is the gel concentration and U⁰_ri. is the relative mobility of component i whrn C = 0. For a given gel concentration, there will usually be some molecular weight range in whiich log M_i and U_ri are approximately linearly related. One typical type of molecule that could approximate this kind of behavior is a long rod, which charge proportional to its length, e.g., DNA. The frictional coefficient of a thin rod of length L is given by

f ~ 3phL/ln(L/b)

If the charge z is proportional to the length L, we get,

U ~ KL/f = (K/3ph)ln(L/b)

At large value of L/b, U will change very slowly with increasing L.

[Example]

Instrumentation

An electrode apparatus consists of a high-voltage supply, electrodes, buffer, and a support for the buffer such as filter paper, cellulose acetate strips, polyacrylamide gel, or a capillary tube. Open capillary tubes are used for many types of samples and the other supports are usually used for biological samples such as protein mixtures or DNA fragments. After a separation is completed the support is stained to visualize the separated components.

Resolution can be greatly improved using isoelectric focusing. In this technique the support gel maintains a pH gradient. As a protein migrates down the gel, it reaches a pH that is equal to its isoelectric point. At this pH the protein is neutral and no longer migrates, i.e., it is focused into a sharp band on the gel.

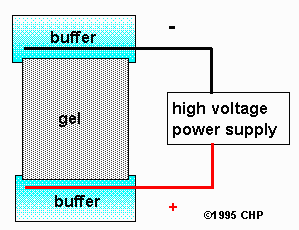
Schematic of zone electrophoresis apparatus

[Chromotography]